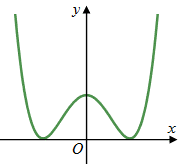
Cho hàm số \[y = f\left( x \right)\]có đạo hàm trên \[\mathbb{R}.\]Đồ thị hàm số \[y = f'\left( x \right)\]như hình vẽ.
Hàm số \[y = f\left( {{x^2} + 2x} \right)\]đồng biến trên khoảng nào sau đây?
Giải bởi Vietjack
Lời giải
Chọn A
Từ đồ thị của hàm số \[y = f'\left( x \right)\]ta có bảng biến thiên của hàm số \[y = f\left( x \right)\]như sau
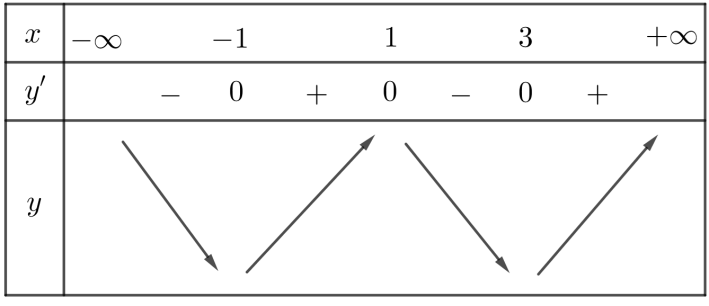
Đặt \[g\left( x \right) = f\left( {{x^2} + 2x} \right)\], ta có \[g'\left( x \right) = {\left( {{x^2} + 2x} \right)^\prime }.f'\left( {{x^2} + 2x} \right) = 2\left( {x + 1} \right).f'\left( {{x^2} + 2x} \right).\]
Hàm số \[g\left( x \right)\]đồng biến khi \[g'\left( x \right) \ge 0 \Leftrightarrow \left( {x + 1} \right).f'\left( {{x^2} + 2x} \right) \ge 0\]
\[ \Leftrightarrow \left\{ \begin{array}{l}x + 1 \ge 0\\f'\left( {{x^2} + 2x} \right) \ge 0\end{array} \right.\quad \left( 1 \right)\]hoặc \[\left\{ \begin{array}{l}x + 1 \le 0\\f'\left( {{x^2} + 2x} \right) \le 0\end{array} \right.\quad \left( 2 \right)\]
·Xét \[\left( 1 \right) \Leftrightarrow \left\{ \begin{array}{l}x + 1 \ge 0\\\left[ \begin{array}{l} - 1 \le {x^2} + 2x \le 1\\{x^2} + 2x \ge 3\end{array} \right.\end{array} \right.\quad \Leftrightarrow \left\{ \begin{array}{l}x \ge - 1\\\left[ \begin{array}{l} - 1 - \sqrt 2 \le x \le - 1 + \sqrt 2 \\x \le - 3\\x \ge 1\end{array} \right.\end{array} \right. \Leftrightarrow \left[ \begin{array}{l} - 1 \le x \le - 1 + \sqrt 2 \\x \ge 1\end{array} \right..\]
·Xét \[\left( 2 \right) \Leftrightarrow \left\{ \begin{array}{l}x + 1 \le 0\\\left[ \begin{array}{l}{x^2} + 2x \le - 1\\1 \le {x^2} + 2x \le 3\end{array} \right.\end{array} \right.\quad \Leftrightarrow \left\{ \begin{array}{l}x \le - 1\\\left[ \begin{array}{l}x = - 1\\\left\{ \begin{array}{l}{x^2} + 2x - 1 \ge 0\\{x^2} + 2x - 3 \le 0\end{array} \right.\end{array} \right.\end{array} \right.\]
\[ \Leftrightarrow \left\{ \begin{array}{l}x \le - 1\\\left[ \begin{array}{l}x = - 1\\\left\{ \begin{array}{l}\left[ \begin{array}{l}x \le - 1 - \sqrt 2 \\x \ge - 1 + \sqrt 2 \end{array} \right.\\ - 3 \le x \le 1\end{array} \right.\end{array} \right.\end{array} \right. \Leftrightarrow \left[ \begin{array}{l} - 3 \le x \le - 1 - \sqrt 2 \\x = - 1\end{array} \right..\]
đồng biến trên khoảng 
. Số phần tử của S là
